V
-
Value in Six Sigma.
All things related to value are touchstones in Six Sigma. Value is essentially how useful a process, product, or service is. How valuable, or how much it is worth, usually depends on its usefulness to the customer. What the customer values, manufacturers strive to produce so they can meet demand. Organizations and Six Sigma practitioners typically use value stream mapping to understand the relationship between product and customer better. This enables them to predict future product demand so they can continue to satisfy customers.
- In Six Sigma, we use the equation value = function / cost. Therefore, the value of a product or service is the exchange for which the customer pays. It also equates more to quality than cost in most circumstances. For example, the higher quality of an item warrants a lower cost for a real-world context. Furthermore, the higher the quality, the greater the cost of improving quality. Although, in the long-term, costs such as those linked to hidden opportunities reduce continuously. For something to be labeled as a value-added process or action, it must correspond to the following conditions. Firstly, the customer should be willing to pay for the action. Secondly, it must be done without error the first time. Thirdly, the action must have a worthwhile effect on the product or service.
W
-
Weighted Moving Average.
WMA, or weighted moving average, is a type of variation that appears in Six Sigma work. WMA is the variation of your moving average (a technique used to eliminate turbulence from data, to identify underlying trends). It appears when you label each of the periods considered with a different weight. The most recent periods tend to receive a greater weight. For instance, imagine a three-period moving average.
- We could display it as so: Fn = 0.5xn-1 + 0.3xn-2 + 0.2xn-3.
- Your forecast for the period n here is the result multiplied by 0.5 for the period n-1. We then add 0.3, the result for n-2, plus 0.2, the previous result. Each weight must be sum to one for weighted moving average. Six Sigma practitioners will be likely to use weighted moving average in statistical work, especially when dealing with turbulent data. Any industry from manufacturing to software development to call centers deal with data of this variety. Six Sigma is inherently reliant on statistical analysis, accurate raw data playing a vital role in the work Six Sigma Belts carry out. Green Belts and Black Belts, even Yellow Belts, may be required to work with WMA.
X
-
XmR Control Charts.
XmR Control Charts are also known as ImR (Individual and Moving Range) Control Charts. Six Sigma Black Belts or Green Belts may use them in statistical and logistical analysis. XmR Control Charts allow you to identify a moving range between consecutive samples. You can use them when larger subgroups become hindered by logistical factors. However, it is more common to use them without a basis for rational subgroups. You can create your XmR or ImR Control Chart by inputting your relevant equations opposite boxes labeled Upper Control Limit and Lower Control Limit. In the same table, below these two boxes and columns, should be a separate area named Moving Chart Range, with corresponding boxes and equations for Upper Control Limit and Lower Control Limit below.
- You can use control charts like these to monitor outputs for your processes. They are useful for providing a prompt warning for ‘special causes’ before they enter a process. All control charts comprise an upper and lower Control Limit, represented, in standard Control Charts, by a through line on the graph. Any points plotted outside of these limits are known as ‘special ‘
Y
-
Yield.
In Six Sigma, yield is a traditional estimate of process performance. You can calculate yield using the following equation: Yield = Output / Input = 100% [your scrap rate]. Here’s an example of how to calculate yield if you had 20 parts with randomly occurring critical errors in a 400-strong sample. Here your scrap rate would equal 20 / 400 multiplied by 100%, which equals 5%. Therefore, your yield would equal 95%. Most Six Sigma practitioners prefer to use throughput yield, an alternate method that often produces better results.
- Throughput Yield measures how capable a process is at producing units free of defect. You should calculate your throughput yield calculated according to how many defects appear per unit (DPU). Throughput Yield is more effective than the standard yield metric, as it deals with the actual number of defective units. This contrasts with the standard yield metric which only considers the total defects for each of your defective units. Additionally, the standard yield estimate tends only to consider the defects which the customer receives. It often ignores the defects that you correct or those that result from inter-business waste.
- Imagine you had to find 60 randomly occurring errors for six critical characteristics on 20 orders in a 400-strong sample. The rate of defect would equate with the percentage of units with one or more defects. For 20 orders with one or more critical defects, your defective rate would be 5%, given the sample of 400. You would follow this calculation: 20 defective orders / 400 units – 0.005. This would also give you a yield of 95% and your defects per unit (DPU) would mean 60 defects / 400 units equals 0.15 or 15%. Here your throughput yield (Yt) would equal 0.85%, meaning an average of 85% of units will be free of defect.
Z
-
Z-Test.
One of many hypothesis tests in Six Sigma, the z-test allows you to test the mean of a specific value. You should use the z-test when you are aware of standard deviation, or if your sample is particularly large (30 plus). You should ensure that your population conforms to normal distribution, using the following hypothesis. H0 = the population mean is the same as the value you specified. H1 = the popular mean is either equal to, less than, or greater than your specific value. Your z-test should look like this:

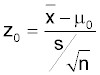








No responses / comments so far.